Various phases such as molten iron,
slag, and gas are all involved in the smelting and refining of
iron and steel. Although some of these phases may consist of
a single substance or compound, they are in many cases a homogeneous
phase composed of multiple components, namely a solution. The
extensive quantities such as the free energy, enthalpy, and entropy
of a solution undergo changes depending on the quantity and composition
of the solution, as well as on its temperature and pressure.
However, owing to interaction among the atoms of the solution
components, the way in which change actually occurs is not as
simple as when elements or compounds are mechanically mixed.
Therefore, the partial molar quantity defined in Eq.1 is used
to represent various thermodynamic relationships for the extensive
quantities of the solution.
The suffixes for the partial differential coefficient in Eq.1
show that they are invariable.  i can
be regarded as the amount of change in the quantity M when only
component i is added in an extremely small quantity at
a constant temperature and constant pressure, without changing
the quantities of the other components. This is defined as the
partial molar quantity of component i. i can
be regarded as the amount of change in the quantity M when only
component i is added in an extremely small quantity at
a constant temperature and constant pressure, without changing
the quantities of the other components. This is defined as the
partial molar quantity of component i.
Among other partial molar quantities, the partial molar Gibb's
energy is important in discussing the activity, etc. of a solution.
It is called the chemical potential and is expressed by 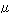 as shown in Eq. 2. as shown in Eq. 2.
The difference between the partial molar quantity of a component
of a solution and the quantity for the pure state of the component
is referred to as the relative partial molar quantity of that
component, and is expressed as Eq. 3.
Equilibrium of the chemical reactions related to a solution is
governed by the activities of these reaction components. Therefore,
knowledge of the activities of these components is necessary
when considering the equilibria of reactions in smelting and
refining and the reactivity of solution components. The activity
of a solution component is defined in Eq. 4 as the ratio of the
fugacity in the solution component to the fugacity in the standard
state of that component (i.e., the ratio of the vapor pressures
of the component when its vapor pressure is low).
The reference and standard state for activity can be arbitrarily
selected. In general, the reference and standard state are adopted
as shown in Eqs. 5-a, 5-b and 5-c for the smelting and refining
of iron and steel. The figure shows the relationship between
the activity and concentration of a solution component, A, B
and C representing the standard state of activities in accordance
with the respective reference states of Eqs. 5-a, 5-b and 5-c.
The relationship in Eq. 6 holds between the relative partial
molar Gibb's energy and the activity of an actual solution component.
The activity of a component in actual solution deviates from
Raoult's law or Henry's law, and hence the activity coefficients
defined by Eqs. 7-a, 7-b and 7-c are used to indicate the degree
of the deviation.
For considering the activity and activity coefficient of the
components in a multi-component solution, more detailed knowledge
of the thermodynamics, such as interaction coefficients and interaction
parameters, is required. |
|
 |
 |
 |
|
|